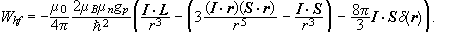sandy_mcd wrote:[..... Since the speed of light is a constant, only two of the these three quantities (speed of light, length unit, time unit) can be arbitrarily defined. In the past, the length unit (e.g., meter) and time unit (e.g., second) were defined. Recently (1983) the meter was redefined in terms of the speed of light.
I had considered pointing out the technical background on how the "second" and the "meter" were defined, but it seems to bring up emotional responses where there shouldn't be any. I haven't checked the relevant Wikipedia entries recently to see the changes, as the contents are somewhat fluid.
I am glad you used the term "arbitrarily defined" as that puts those two units into their proper perspective. The NIST web site presents the definitions of the base units. The NIST historical content on the second doesn't mention the ephemeris second.
http://physics.nist.gov/cuu/Units/current.html
The documents that describe the selection of the ephemeris second are available at the following URL:
http://www.leapsecond.com/history/1958- ... -Parry.pdf
The purpose of assigning 9,192,631,770 cycle counts of the hyperfine transitions (caesium-133) was to provide scientists with a "stable" reference, since the ephemeris second had variations which were plus or minus 20 counts, this based upon astronomical observations some 53+ years ago.
The Swiss Office of Metrology provides official definitions for the base units but they approach it with a little more honesty.
http://www.metas.ch/en/scales/meter.html
The metre definition assigns a fixed value to the speed of light c. This fundamental constant can therefore no longer be measured; it has been fixed by definition. From this can be concluded that the unit of length is dependent on the unit of time, the second.
Now we are down to one unit that has been arbitrarily defined, the second. The duration of the second is based upon an astronomical observation peculiar to the earth, and I have a hard time considering this the basis for a system of units which is being used to try to understand the "nature of the universe". All the SI "derived units" are defined using the base units.
sandy_mcd wrote:It is important to distinguish between "accuracy" and precision". ...
In my last post I mentioned "significant figures" in relationship to the speed of light and "precision" in relationship to counters. The speed of light value is calculated using two transcendental numbers thus it can never be absolutely precise, but I wouldn't call 5+ million significant figures inaccurate. Our ability to calculate the number of significant figures is limited only by our computer technology.
sandy_mcd wrote:The factor of 10 to the 6th is just randomly thrown in.
Not really. When the length 47.713 cm is converted to a frequency it has the value of 628.31 (10<sup>6</sup>). The whole premise of the geometric, wavelength and frequency relationships is based upon electromagnetic values which are typically expressed in megacycles, or megaHz using the Hertz nomenclature.
sandy_mcd wrote:Energy levels in atoms still need to be measured with respect to some energy scale. ..
I couldn't agree more. The following URL, Node11.html, denotes the energy differences within the various energy splittings of the hydrogen atom. Node9.html is specific to the hyperfine transition.
http://www.pha.jhu.edu/%7Ert19/hydro/node11.html
http://www.pha.jhu.edu/%7Ert19/hydro/node9.html
In equation (86) in the node9 article, the calculation uses variety of symbols representing "units", including c<sup>2</sup>, to determine the energy level in each state (f=0, f=1), and the separation between the two states gives the 5.9 (10<sup>-6</sup>) eV value. We know that the numeric value of the speed of light is based upon "arbitrary" units. The eV (electron volt) value is based on the joule, and the joule is based upon the "newton" and the "meter". We know where the meter comes from, and the newton is based upon the SI definition for a kilogram and a time of one "second", and we know where the second comes from. The kilogram is based upon a physical object, and its selection was very arbitrary. Most of the unit definitions are in the dictunit.htm page. The eV definition is in the Wolfram page.
http://www.ex.ac.uk/cimt/dictunit/dictunit.htm
http://scienceworld.wolfram.com/physics ... nVolt.html
If all energy levels are referenced to the frequency of the hydrogen hyperfine transition emission, using the mathematically derived value, you can eliminate all the "arbitrary " units. The "unit of energy" would have a natural relationship to the "unit of time", the "unit of length" and the "speed of light". What would we call the "unit of energy" represented by a frequency of 888.5765876.... (10<sup>6</sup>)? We know imperically that this "unit of energy" will be a very small value, so we might consider randomly throwing in a 10 to the minus 6 scaling factor.
sandy_mcd wrote:It is this ~ 21 cm line which the author propose as a new unit of length. The proposed unit of length does not simplify much as it is a quantity derived from more basic constants. To see what would be more natural, see
http://en.wikipedia.org/wiki/Natural_units. In these examples, values of certain basic constants are defined in order to make some equations simpler or more elegant.
I've been through that wiki article before and it looks like they have added "Stoney Units", which I hadn't heard of before. The "simplified" units do make the equations simpler and seemingly elegant, but when they want to apply "real world" numeric results they still have to convert to SI units, which are burdened with "arbitrary" units. You might want to look at the superstring site for a somewhat simpler description of "Natural Units" used by particle physicists.
http://superstringtheory.com/unitsa.html
We know that scientists that created the various "natural units", where c is equated to "1", did so because the current numeric value is unwieldly and is arbitrary because of the base units. I suspect everyone of those scientists would not hesitate to include the symbols representing the values for the two constants (square root of 2, and 2 pi) in their formulas because they know that many phenomena in the physical world have Pi relationships embedded within them.
One of the committees that provides recommendations to the SI organization has stated this,
iupap wrote:- the consensus that now exists on the desirability of finding ways of defining all of the base units of SI in terms of fundamental physical constants so that they are universal, permanent and invariant in time;
http://www.iupap.org/commissions/interu ... 1-2005.pdf
I doubt that they will succeed until they replace the arbitrary value used for the "duration" of the second with a value that is mathematically derived.
sandy_mcd wrote:The origin of this idea can be found at
http://www.vip.ocsnet.net/~ancient/Universal.pdf on page 1. The more I read, the odder it sounds. It seems that the author noticed that some trigonometric value for some triangle coincidentally matched the digits for the hyperfine splitting in the H atom and went off from there.
The Universal.pdf page was one of my first attempts to write down the mathematical characteristics of the geometric electromagnetic relationships. I had not identified the "simple" trigonometric relationships expressed in the EuclideanUnits-TheBasics.pdf page at that time.
Yes, there is an "oddity" in how I found the triangle relationship that used the H atom hyperfine wavelength. I was not looking for it, but it was the eventual result of reading a book that I thought was going to be some casual Winter reading. The book has a strong religious connotation and because of that I do not identify it. If you haven't noticed, the discussion of religion can create strong emotional responses, and I knew this would automatically turn people "off" if I mentioned it such they would never examine the mathematics. The original author of the material included in the book I read did not know what he had, he simply recorded a great many observations, some not originally his. It was not a technical book. I had some familiarity with electromagnetic principles and realized that things stated in the book "did not fit". It took me about a month to gather additional references that covered the same material by different authors and then "fill in" the missing pieces.
Hamming wrote:As always happens when I become involved in the topic, I again came away with the feeling that "God made the universe out of complex numbers."
I would like to paraphrase Hamming and add, "God made the universe out of transcendental numbers, they go on forever, never ending."
The last paragraph of the Universal.pdf article is a quotation,
Maxwell wrote:The most universal standard of length which we could assume would be the wavelength of a particular kind of light... Such a standard would be independent in any changes in the dimension of the earth, and should be adopted by those who expect their writings to be more permanent than that body. James Clerk Maxwell, 1873
Maxwell made that statement in regards to the controversies at that time related to whether British scientists should accept the meter as an official length.
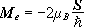 and
and 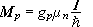 and
and  we have
we have